Valuing Private Equity Strip by Strip
Using Synthetic Dividend Strips for Asset Pricing in non-Listed Securities
A paper of mine with Stijn Van Nieuwerburgh: “Valuing Private Equity Strip by Strip” is now forthcoming at the Journal of Finance, so I’d like to write about this project and ways I hope other people can extend our approach.
Asset Pricing with Prices
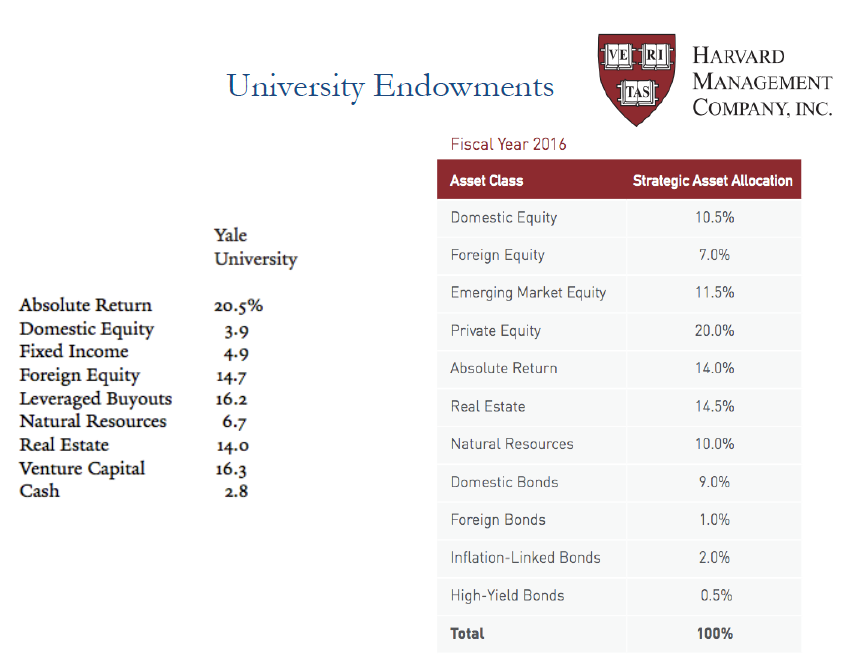
It’s really important to figure out how to measure the risk and return of non-listed investment vehicles like Private Equity and Venture Capital. These kinds “alternative” assets like these have really taken over the portfolios of many institutional investors. You can see Yale and Harvard’s endowment, for instance, is so heavily in this kind of stuff while the “traditional” securities like stocks and bonds actually just make up a tiny fraction of the overall portfolio:
These asset categories work in a somewhat opaque manner. As the investor (LP), you offer the manger (the GP) a capital commitment. The GP calls the capital over time, invests it in different companies or assets, and then distributes back this cash back to you according to a waterfall taking into account sizable fees. So you get a stream of cash that will look like this across fund life, depending on the precise fund category you invest in:
What you want to know as an investor is — well, did I do poorly or well? Sure, I invested some money and got a bunch back. But what kind of risk did I take in the process? Did I outperform on a risk-adjusted basis, or was I really just paying for some kind of risk factor exposure?
With publicly listed assets, for which we have a liquid price to can look at, Asset Pricing has a standard playbook — you regress the returns against some series of factors (canonically the Fama-French 3-factor model but there are others) which represent payoffs on investment portfolios. You get the beta or risk loadings on these factors, which tells you the risk exposure of your investment, as well as your alpha — how much your investment outperformed on a risk-adjusted basis.
Asset Pricing without Prices
It’s hard to do something similar with these PE investments (and with private investments generically); since we don’t have a liquid price of the investment. In fact, the lack of a liquid price is sort of the whole point of the asset class which I’ll come back to. This makes it really hard to tell whether alternative asset investments which institutional investors are doing makes sense; or what risk they are actually taking.
Now there is some prior work that we build on here. The PME idea by Steve Kaplan and Antoinette Schoar evaluates PE by comparing a $1 investment in a PE fund with a $1 invested in the S&P 500 as a counterfactual portfolio with a beta = 1. Arthur Korteweg and Stefan Nagel extend this idea to consider the GPME — which allows for an arbitrary beta or loading on the public market in risk-adjusting VC funds.
We create a new general methodology that can value any generic streams of cashflows including those that are not publicly traded. The basic intuition is that instead of regressing on returns, we match the cash that PE firms return against the cash produced by a series of replicating assets across horizon and risk category. These assets — synthetic dividend and capital gains strips — correspond to the payoffs to holding certain factor strategies (like growth, value, etc. just as in Fama-French) but for specific maturities (ie 1 year, 5 years, 10 years).
So a value dividend strip, for example, will pay out cash from a strategy that holds and harvesting the dividends on a particular horizon (say, 5 years out). Owning value stocks, overall, just gives you the claims to value dividend strips across all horizons. A value capital gains strip is going to reflect the returns to buying, holding, and selling value stocks at a certain time. This horizon dimension is just important because PE has a finite horizon, while stocks are very long-duration, so you want to line up the cash flows appropriately.
This gives us something like fundamental Arrow-Debreu securities, and I think these dividend and capital gains strips are nice way to think about measuring risk and return on a basic level.
We have a public GitHub page which contains dividend strip data, code, some synthetic PE data. And a coding video here which walks you through the data and risk-measurement process. Part of the goal is to create a general platform other researchers can use to explore risk and return characteristics elsewhere, and these resources should help you get started:
Private Equity as a Negative Alpha Asset
Okay, so back to these dividend strips. Isn’t that similar to something that Jules van Binsbergen, Michael Brandt, and Ralph Koijen looked at, you ask?
So yes — they looked at these publicly traded dividend strips which paid dividends on specific horizons, but not further. This was a really nice discovery which helped people think about the term structure of equity returns — what the price of equity risk looks like across maturities for the market as a whole. Our project creates synthetic dividend strips for a broad range of factors across a longer timespan.
The core of the project is figuring out how to regress these dividend and capital gains strips against Private Equity funds across a lot of different categories (standard Buyout; VC, Real Estate, etc.). You can do this in a standard OLS framework, but it gets complicated when we have a large number of possible factors and many horizons. This is the “factor zoo” world, and we use ML techniques in the form of an elastic net to do a shrinkage estimation. This imposes a LASSO penalty (which just zeros out a bunch of exposures) as well as a ridge regression (shrinking some exposures).
In the end this just tells us what the replicating portfolio for PE funds looks like, which helps to open up that “black box” and tell us what the underlying risk is. For VC, for example, we find a good amount of growth gains strips for the estimated exposure. This makes sense — returns to VC can be replicated by buying, holding, and selling publicly traded growth stocks. And similarly Real Estate funds take on exposure proxied by public REITs. While intuitive, our approach just tells you exactly how much growth or REIT exposure you need to replicate these funds.
So we’ve figured out exactly how risky these vehicles are! This opens the door for a lot of interesting things — one of which in particular is the alpha. How much do PE vehicles deliver to investors, on a risk-adjusted basis?
We find negative alpha across the board when we allow for a broad mix of possible factors. Here are a couple of profit distributions for funds comparing standard PE Buyout funds and VC (the yellow histogram is our full factor model). Buyout delivers investors an additional $0.62 per $1 of committed capital; but this winds up being a -$0.06 alpha taking into account the risk the funds are taking. This result is robust to the specific choice of dataset and these risk-adjusted profits (RAPs) are decreasing over time.
The Illiquidity Premium and Phony Happiness
Our results make sense in the broader context that others have found that risk-correction also lowers alpha for other active asset managers like hedge funds and mutual funds. Our results don’t imply that PE doesn’t create any value, to be clear. But they are consistent with a Berk and Green story in which the value is extracted as fees by the GPs, leaving little in the way of excess return for the investors.
This is a little surprising in PE, though, because you might imagine that locking up capital for investment purposes is less desirable by investors, who demand a premium for this feature. Universities with large PE portfolios, after all, were stuck with these illiquid investments during the Covid crisis and could not liquidate to meet immediate cash needs. The whole idea of this investing style, going back to David Swensen who really expanded alternatives investment for endowments, is that long-lived institutions like Universities should be able to harvest these gains from holding illiquid assets including exotic assets like forests and ride out market turmoil.
Our results — and those of others who also find little excess performance of PE — are more consistent with another theory. As Cliff Asness suggests: it could be illiquidity that commands a premium. Instead of PE as being this exotic asset class which offers excess returns in exchange for opacity — maybe the opacity itself is the main benefit. Being able to access returns away from a volatile public market is sufficiently valuable, in this view, that investors actually pay for the benefit of not knowing the true price. This is also a perspective you will find in other market participants; Gavin Baker here for instance:


You also hear this from public pensions, who are major institutional investors in PE. The CIO of an Idaho pension plan for example:
We did know that our actuaries and accountants would accept the smoothing that the accounting would do. It may be phony happiness, but we just want to think we are happy," he said. "If [private equity] just gave public market returns, we’d be in favor of it because it has some smoothing effects on both reported and actual risks.
Extending Asset Pricing
The “illiquidity discount” view remains controversial, but I think it helps to explain why so many institutional investors have been pilling into this asset class despite unclear returns. The broader point here though is that we have put together a general purpose technology that helps us understand the basic building blocks of risk that make up institutional investor portfolios, so that we can have these kinds of informed discussions about whether these alternative asset investing strategies make sense.
And our work here is open source. So please take the dividend and capital gains strips and see how well you can do at valuing private firms, oil wells, infrastructure projects, or anything else; and let me know what you find. Thanks as well to the editor, Stefan Nagel, and two very informed and helpful referees at the JF.






Excellent post and congrats on the JF! This is very useful for the M&A course I teach which covers buyouts and PE funds.